Ars Combinatoria
This section contains as yet unpublished manuscripts by Leibniz that concern the Ars Combinatoria and more particularly his tabular methods. The German philosopher's combinatorial practice is based on two tools: the table, which Leibniz links to inventories, and thus to the Ars Inveniendi, and the form, which he links to the characteristic, and thus to the Ars Characteristica. The table and the form are in fact two sides of the same coin: one develops and the other envelops. Indeed, the table is a tool that allows Leibniz to arrange different terms in bidimensional space. The relations between these terms then establish a synoptic panorama of the different possible combinations and the way they are generated. The form, on the other hand, envelops all the combinations within it. The combinatorial structure is expressed by means of the identical. Indeed, the equality between two forms expresses the invariants that define the combinatorial subclasses of a structure.
It is through the tabular practice that combinatorics is first integrated within Leibnizian mathematics, at the beginning of the Parisian stay. These works, published in the third volume of the Akademie's series VII, deal with questions of series and quadratures. From 1674 onwards, Leibniz worked intensively on algebraic questions and the tables gradually took on a purely heuristic role in his work.
The texts we propose here are texts from after the Paris period (1677-1680), in which Leibniz uses tables to analyse problems related to series and quadratures, despite his considerable interest in formal aspects and symbolic problems at that time. These texts constitute a double counterweight in the image that one tends to construct of Leibniz's mathematical practice. On the one hand, Leibniz uses diagrams as a tool for calculation and not only as a support for the imagination, which should be distrusted. On the other hand, he is an experimental mathematician who gives great importance to induction and does not resign himself to reducing all reasoning to a mechanical and deductive derivation.
Overviews
Overview of De summis serierum Arithmo-Geometricarum infinitarum
Leibniz wrote this text in January 1678, i.e. a period shortly after his departure from Paris when questions about the analysis of progressions were gaining in interest. The problem Leibniz wanted to solve was to find the sum of what he called arithmo-geometric series: $0^p.t + 1^p.t^2 + 2^p.t^3 + \dots + (n-1)^p.t^n + \dots$ The terms of the series are composed of two factors, one of which, $t^n$, follows a law of geometric progression, and the other $n^p$ follows an arithmetic law, raised to a certain power $p$.
viewOverview of Tabula Numerorum Arithmeticae Replicationis seu Figuratorum interpolata
This manuscript is a copy of the interpolation table found in Wallis' Arithmetica Infinitorum of 1656, at
proposition 135, p.165.
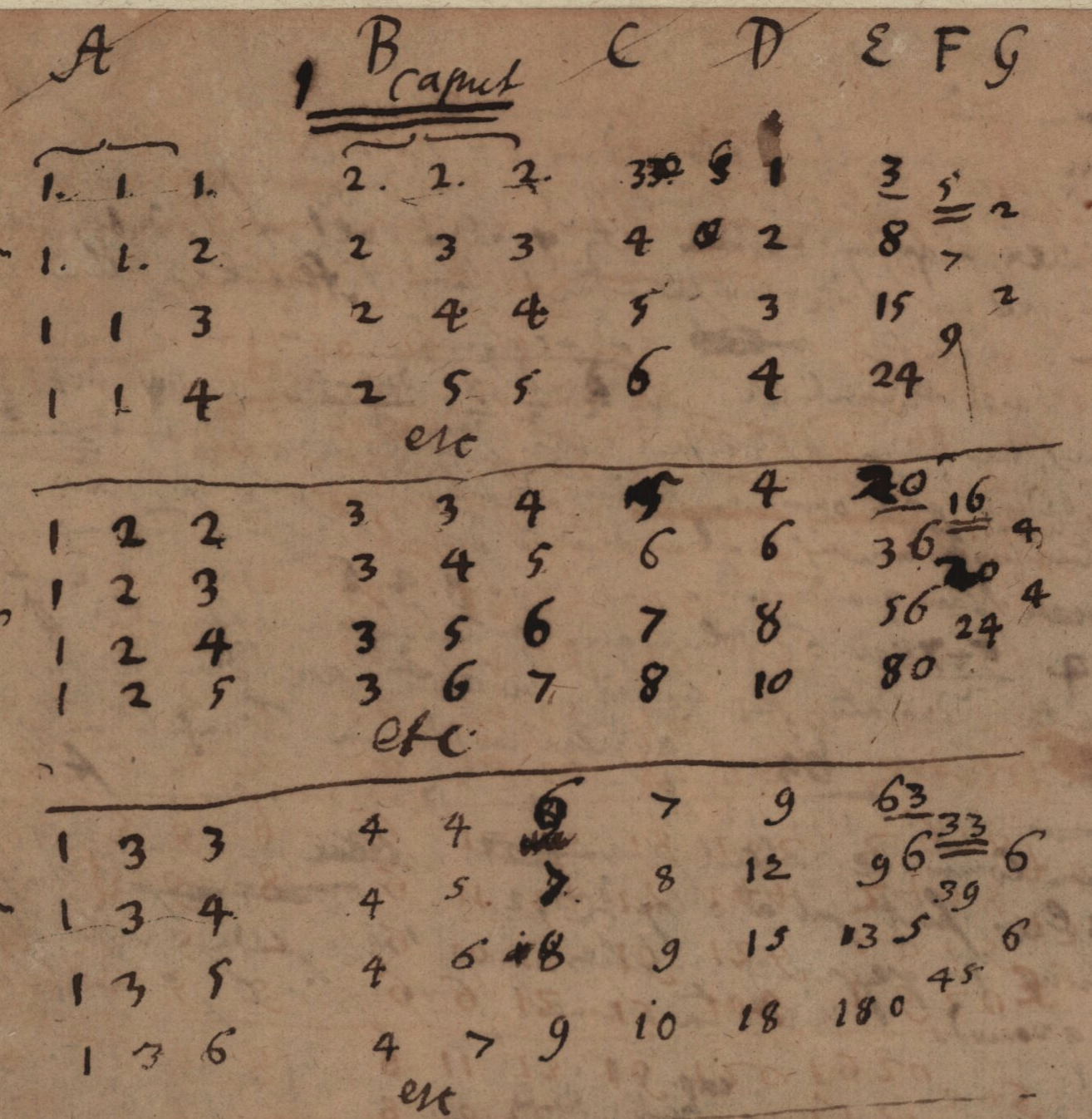
Overview of Untitled (Tables sur la formule de Heron)
This manuscript is dated March 1678. It is part of a corpus of texts in which Leibniz works on diophantine problems, in particular triangles in numbers, i.e. triangles whose sides all have integer ratios to each other. Here, Leibniz thus engages in a systematic study of this problem, developing a method for generating all possible triangles in numbers. However, isolating this manuscript from the arithmetical context in which it appears to be set, Leibniz's presentation of it places it more within the framework of geometry, and more particularly geodesy. In mentioning this discipline, Leibniz refers directly to Heron of Alexandria, whose famous formula for determining the area of any triangle from its three sides is being studied. Thus, this manuscript must be situated at the crossroads of research on practical mathematics and applied geometry and those interested in number theoretical problems.
viewOverview of Untitled, sur l’interpolation de Mengoli
This text is part of a wider investigation by Leibniz in 1679 into the question of transcendence. On this occasion, he reopened a file that he had probably closed at the end of his stay in Paris in 1676: the interpolation techniques of Wallis and Mengoli for squaring the circle.
On the front are two triangular diagrams derived from Mengoli's triangular tables, which are probably copies of the diagrams that Leibniz wrote in his 1676 notes on Mengoli's Circolo. In the left-hand margin of the triangular tables, there is a geometric description of the curves represented by each term of the triangle. In the right-hand margin, Leibniz compares the advantages of this tabular method with those of Newton's formula.
